Draw a diagram of an AC generator and describe it. Derive an expression for instantaneous value of induced emf.
Hint: An A.C. This generator is an electronic apparatus designed to transform mechanical energy into electrical energy through the principle of electromagnetic induction. Create a diagram by conducting a schematic analysis of its operation, ensuring to include all key components. The formula for induced electromotive force (emf) can be derived using the equation for magnetic flux in the coil and Faraday’s law of induction.
Formula Used:
Magnetic flux across the coil, \(ϕ=NBAcosθ\)
Faraday’s flux rule, \(ε=−\frac{dϕ}{dt}\)
Complete step-by-step answer:
A generator, or dynamo, is a device that transforms mechanical energy into electrical energy. The term “generator” is somewhat misleading, as it doesn’t actually create energy; instead, it merely changes energy from one form to another.
An A.C. A generator is a device that generates an alternating current, meaning it reverses its direction at regular intervals. This device transforms mechanical energy into a fluctuating type of electrical energy. The operation of AC generators is based on the principle of electromagnetic induction. When a closed coil is spun in a uniform magnetic field with its axis perpendicular to the field, the magnetic flux associated with the coil changes, inducing an electromotive force (emf) and, consequently, a current within it.
The different parts of an A.C. generator include:
- Field magnet
- Armature
iii. Slip rings
- Brushes
- Source of energy
The following diagram shows an A.C. generator:
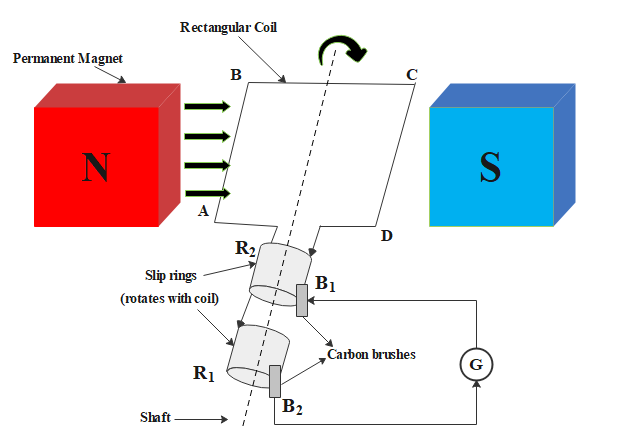
The expression for instantaneous value of induced emf:
Consider the number of turns in the coil of the A.C. generator are N, its face area for each turn is A, the magnitude of the magnetic field is B and θ is the angle which normal to the coil makes with magnetic field B at any instant t. Let the angular velocity with which the coil rotates be ω.
At any moment t , the magnetic flux associated with the coil of the A.C. generator can be expressed as:
\(ϕ=NBAcosθ\) or,
\(ϕ=NBAωt\)
According to Faraday’s law of flux, the induced emf can be expressed as:
\(ε=−\frac{dϕ}{dt}\)⇒\(ε=−\frac{d}{dt}\)(NBAcosωt)
⇒\(ε=NBAωsinωt\)
\(∴ε=ε_∘sinωt\)where\(ε_∘=NBAω\). This equation gives the expression for instantaneous value of induced emf.
Note: The generation of A.C. is more economical than the generation of D.C. Furthermore, the alternating voltage can be easily increased or decreased to meet the circuit’s requirements using a transformer.


